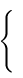ORIGIN CHOICE 1, Origin at -4m 2 at -4/n m 2/g, at -1/4, 1/4, 0 from centre (2/m)
Generators selected (1); t(1, 0, 0); t(0, 1, 0); t(0, 0, 1); (2); (3); (5); (9)
Multiplicity, Wyckoff letter,
Site symmetry | Coordinates |
|
| | |
| | (1) x, y, z | (2) -x, -y, z | (3) -y + 1/2, x + 1/2, z | (4) y + 1/2, -x + 1/2, z | | (5) -x + 1/2, y + 1/2, -z | (6) x + 1/2, -y + 1/2, -z | (7) y, x, -z | (8) -y, -x, -z | | (9) -x + 1/2, -y + 1/2, -z | (10) x + 1/2, y + 1/2, -z | (11) y, -x, -z | (12) -y, x, -z | | (13) x, -y, z | (14) -x, y, z | (15) -y + 1/2, -x + 1/2, z | (16) y + 1/2, x + 1/2, z |
|
I Maximal translationengleiche subgroups
| [2] P-4m2 (115) | 1; 2; 7; 8; 11; 12; 13; 14 |
|
|
| [2] P-421m (113) | 1; 2; 5; 6; 11; 12; 15; 16 |
|
|
| [2] P4mm (99) | 1; 2; 3; 4; 13; 14; 15; 16 |
| 1/2, 0, 0
|
| [2] P4212 (90) | 1; 2; 3; 4; 5; 6; 7; 8 |
|
|
| [2] P4/n11 (85, P4/n) | 1; 2; 3; 4; 9; 10; 11; 12 |
|
|
| [2] P2/n12/m (67, Cmme) | 1; 2; 7; 8; 9; 10; 15; 16 | a - b, a + b, c
| 1/4, 3/4, 0
|
| [2] P2/n21/m1 (59, Pmmn) | 1; 2; 5; 6; 9; 10; 13; 14 |
|
|
II Maximal klassengleiche subgroups
[2] c' = 2c
| P42/ncm (138) | <2; 9; (3; 5) + (0, 0, 1)> | a, b, 2c | 0, 0, -1/2 |
| P42/ncm (138) | <2; 5; (3; 9) + (0, 0, 1)> | a, b, 2c | |
| P42/nmc (137) | <2; 5; 9; 3 + (0, 0, 1)> | a, b, 2c | 0, 0, -1/2 |
| P42/nmc (137) | <2; (3; 5; 9) + (0, 0, 1)> | a, b, 2c | |
| P4/ncc (130) | <2; 3; 9; 5 + (0, 0, 1)> | a, b, 2c | |
| P4/ncc (130) | <2; 3; 5; 9 + (0, 0, 1)> | a, b, 2c | 0, 0, 1/2 |
| P4/nmm (129) | <2; 3; 5; 9> | a, b, 2c | |
| P4/nmm (129) | <2; 3; (5; 9) + (0, 0, 1)> | a, b, 2c | 0, 0, 1/2 |
[3] c' = 3c
 | P4/nmm (129) | <2; 3; 5; 9> | a, b, 3c | | | P4/nmm (129) | <2; 3; (5; 9) + (0, 0, 2)> | a, b, 3c | 0, 0, 1 | | P4/nmm (129) | <2; 3; (5; 9) + (0, 0, 4)> | a, b, 3c | 0, 0, 2 |
|
- Series of maximal isomorphic subgroups
[p] c' = pc
| P4/nmm (129) | <2; 3; (5; 9) + (0, 0, 2u)> | a, b, pc | 0, 0, u | | | p > 2; 0 ≤ u < p
p conjugate subgroups for the prime p |
|
[p2] a' = pa, b' = pb
| P4/nmm (129) | <2 + (2u, 2v, 0); 3 + (p/2 - 1/2 + u + v, p/2 - 1/2 - u + v, 0); 5 + (p/2 - 1/2 + 2u, p/2 - 1/2, 0); 9 + (p/2 - 1/2 + 2u, p/2 - 1/2 + 2v, 0)> | pa, pb, c | u, v, 0 | | | p > 2; 0 ≤ u < p; 0 ≤ v < p
p2 conjugate subgroups for the prime p |
|
I Minimal translationengleiche supergroups
II Minimal non-isomorphic klassengleiche supergroups
- Additional centring translations
| [2] C4/mmm (123, P4/mmm); [2] I4/mmm (139) |
ORIGIN CHOICE 2, Origin at centre (2/m) at n 21 (2/m, 21/g), at 1/4, -1/4, 0 from -4 m 2
Generators selected (1); t(1, 0, 0); t(0, 1, 0); t(0, 0, 1); (2); (3); (5); (9)
Multiplicity, Wyckoff letter,
Site symmetry | Coordinates |
|
| | |
| | (1) x, y, z | (2) -x + 1/2, -y + 1/2, z | (3) -y + 1/2, x, z | (4) y, -x + 1/2, z | | (5) -x, y + 1/2, -z | (6) x + 1/2, -y, -z | (7) y + 1/2, x + 1/2, -z | (8) -y, -x, -z | | (9) -x, -y, -z | (10) x + 1/2, y + 1/2, -z | (11) y + 1/2, -x, -z | (12) -y, x + 1/2, -z | | (13) x, -y + 1/2, z | (14) -x + 1/2, y, z | (15) -y + 1/2, -x + 1/2, z | (16) y, x, z |
|
I Maximal translationengleiche subgroups
| [2] P-4m2 (115) | 1; 2; 7; 8; 11; 12; 13; 14 |
| 1/4, 3/4, 0
|
| [2] P-421m (113) | 1; 2; 5; 6; 11; 12; 15; 16 |
| 1/4, 3/4, 0
|
| [2] P4mm (99) | 1; 2; 3; 4; 13; 14; 15; 16 |
| 1/4, 1/4, 0
|
| [2] P4212 (90) | 1; 2; 3; 4; 5; 6; 7; 8 |
| 1/4, 3/4, 0
|
| [2] P4/n11 (85, P4/n) | 1; 2; 3; 4; 9; 10; 11; 12 |
|
|
| [2] P2/n12/m (67, Cmme) | 1; 2; 7; 8; 9; 10; 15; 16 | a - b, a + b, c
|
|
| [2] P2/n21/m1 (59, Pmmn) | 1; 2; 5; 6; 9; 10; 13; 14 |
|
|
II Maximal klassengleiche subgroups
[2] c' = 2c
| P42/ncm (138) | <2; 9; (3; 5) + (0, 0, 1)> | a, b, 2c | |
| P42/ncm (138) | <2; 5; (3; 9) + (0, 0, 1)> | a, b, 2c | 0, 0, 1/2 |
| P42/nmc (137) | <2; 5; 9; 3 + (0, 0, 1)> | a, b, 2c | |
| P42/nmc (137) | <2; (3; 5; 9) + (0, 0, 1)> | a, b, 2c | 0, 0, 1/2 |
| P4/ncc (130) | <2; 3; 9; 5 + (0, 0, 1)> | a, b, 2c | |
| P4/ncc (130) | <2; 3; 5; 9 + (0, 0, 1)> | a, b, 2c | 0, 0, 1/2 |
| P4/nmm (129) | <2; 3; 5; 9> | a, b, 2c | |
| P4/nmm (129) | <2; 3; (5; 9) + (0, 0, 1)> | a, b, 2c | 0, 0, 1/2 |
[3] c' = 3c
 | P4/nmm (129) | <2; 3; 5; 9> | a, b, 3c | | | P4/nmm (129) | <2; 3; (5; 9) + (0, 0, 2)> | a, b, 3c | 0, 0, 1 | | P4/nmm (129) | <2; 3; (5; 9) + (0, 0, 4)> | a, b, 3c | 0, 0, 2 |
|
- Series of maximal isomorphic subgroups
[p] c' = pc
| P4/nmm (129) | <2; 3; (5; 9) + (0, 0, 2u)> | a, b, pc | 0, 0, u | | | p > 2; 0 ≤ u < p
p conjugate subgroups for the prime p |
|
[p2] a' = pa, b' = pb
| P4/nmm (129) | <2 + (p/2 - 1/2 + 2u, p/2 - 1/2 + 2v, 0); 3 + (p/2 - 1/2 + u + v, -u + v, 0); 5 + (2u, p/2 - 1/2, 0); 9 + (2u, 2v, 0)> | pa, pb, c | u, v, 0 | | | p > 2; 0 ≤ u < p; 0 ≤ v < p
p2 conjugate subgroups for the prime p |
|
I Minimal translationengleiche supergroups
II Minimal non-isomorphic klassengleiche supergroups
- Additional centring translations
| [2] C4/mmm (123, P4/mmm); [2] I4/mmm (139) |