| Pc | Cs2 | m | Monoclinic  |
| No. 7 | P1c1 | Patterson symmetry P12/m1 | |
| UNIQUE AXIS b, CELL CHOICE 1 | |||
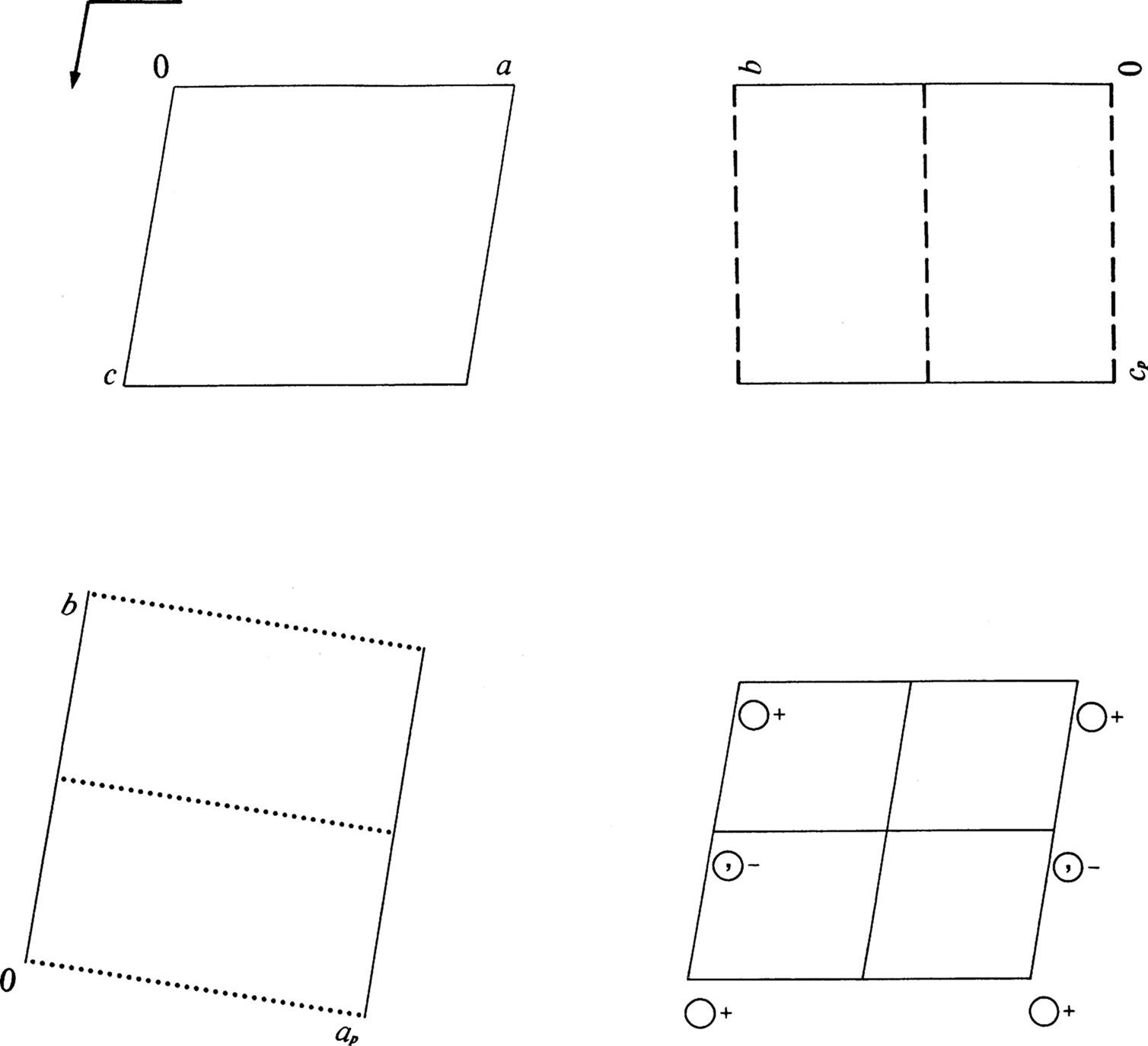
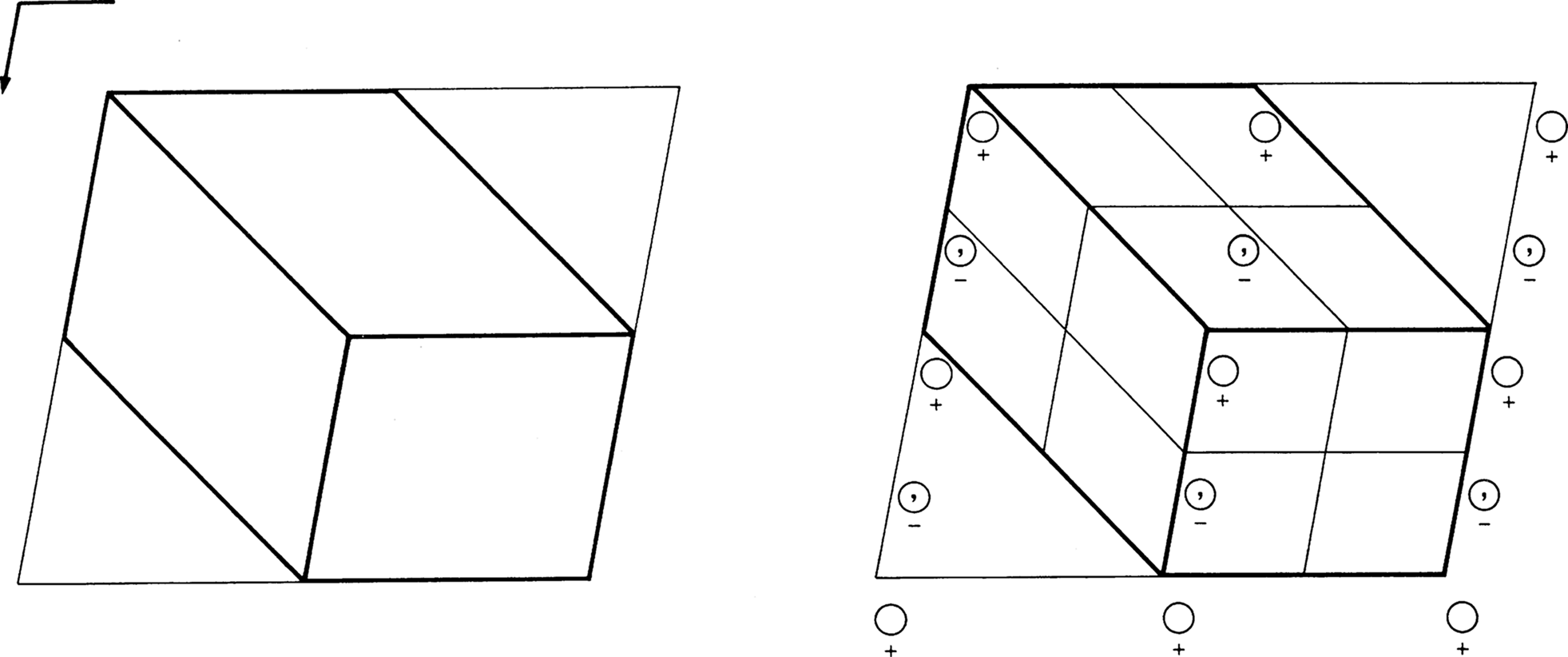
Origin on glide plane c
| Asymmetric unit | 0 ≤ x ≤ 1; 0 ≤ y ≤ 1/2; 0 ≤ z ≤ 1 |
Symmetry operations
| (1) 1 | (2) c x, 0, z |
Generators selected (1); t(1, 0, 0); t(0, 1, 0); t(0, 0, 1); (2)
Positions
| Multiplicity, Wyckoff letter, Site symmetry | Coordinates | Reflection conditions | |||||
| General: | |||||||
|
| h0l : l = 2n 00l : l = 2n |
Symmetry of special projections
| Along [001] p11m a' = ap b' = b Origin at 0, 0, z | Along [100] p1g1 a' = b b' = cp Origin at x, 0, 0 | Along [010] p1 a' = 1/2c b' = a Origin at 0, y, 0 |
Maximal non-isomorphic subgroups
| I | [2] P1 (1) | 1 |
| IIa | none |
| IIb | [2] C1c1 (a' = 2a, b' = 2b) (Cc, 9) |
Maximal isomorphic subgroups of lowest index
| IIc | [2] P1c1 (b' = 2b) (Pc, 7); [2] P1c1 (a' = 2a or a' = 2a, c' = 2a + c) (Pc, 7) |
Minimal non-isomorphic supergroups
| I | [2] P2/c (13); [2] P21/c (14); [2] Pmc21 (26); [2] Pcc2 (27); [2] Pma2 (28); [2] Pca21 (29); [2] Pnc2 (30); [2] Pmn21 (31); [2] Pba2 (32); [2] Pna21 (33); [2] Pnn2 (34); [2] Aem2 (39); [2] Aea2 (41) |
| II | [2] C1c1 (Cc, 9); [2] A1m1 (Cm, 8); [2] I1c1 (Cc, 9); [2] P1m1 (c' = 1/2c) (Pm, 6) |
UNIQUE AXIS b, DIFFERENT CELL CHOICES
P1c1
UNIQUE AXIS b, CELL CHOICE 1
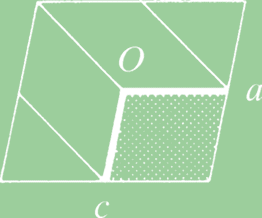
Origin on glide plane c
| Asymmetric unit | 0 ≤ x ≤ 1; 0 ≤ y ≤ 1/2; 0 ≤ z ≤ 1 |
Generators selected (1); t(1, 0, 0); t(0, 1, 0); t(0, 0, 1); (2)
Positions
| Multiplicity, Wyckoff letter, Site symmetry | Coordinates | Reflection conditions | |||||
| General: | |||||||
|
| h0l : l = 2n 00l : l = 2n |
P1n1
UNIQUE AXIS b, CELL CHOICE 2
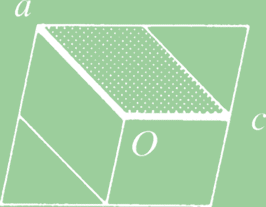
Origin on glide plane n
| Asymmetric unit | 0 ≤ x ≤ 1; 0 ≤ y ≤ 1/2; 0 ≤ z ≤ 1 |
Generators selected (1); t(1, 0, 0); t(0, 1, 0); t(0, 0, 1); (2)
Positions
| Multiplicity, Wyckoff letter, Site symmetry | Coordinates | Reflection conditions | |||||
| General: | |||||||
|
| h0l : h + l = 2n h00 : h = 2n 00l : l = 2n |
P1a1
UNIQUE AXIS b, CELL CHOICE 3
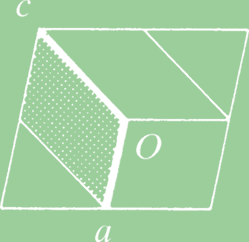
Origin on glide plane a
| Asymmetric unit | 0 ≤ x ≤ 1; 0 ≤ y ≤ 1/2; 0 ≤ z ≤ 1 |
Generators selected (1); t(1, 0, 0); t(0, 1, 0); t(0, 0, 1); (2)
Positions
| Multiplicity, Wyckoff letter, Site symmetry | Coordinates | Reflection conditions | |||||
| General: | |||||||
|
| h0l : h = 2n h00 : h = 2n |
| Pc | Cs2 | m | Monoclinic |
| No. 7 | P11a | Patterson symmetry P112/m | |
| UNIQUE AXIS c, CELL CHOICE 1 | |||
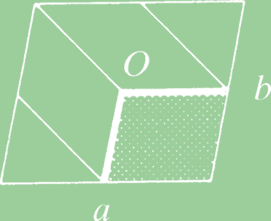
Origin on glide plane a
| Asymmetric unit | 0 ≤ x ≤ 1; 0 ≤ y ≤ 1; 0 ≤ z ≤ 1/2 |
Symmetry operations
| (1) 1 | (2) a x, y, 0 |
Generators selected (1); t(1, 0, 0); t(0, 1, 0); t(0, 0, 1); (2)
Positions
| Multiplicity, Wyckoff letter, Site symmetry | Coordinates | Reflection conditions | |||||
| General: | |||||||
|
| hk0 : h = 2n h00 : h = 2n |
Symmetry of special projections
| Along [001] p1 a' = 1/2a b' = b Origin at 0, 0, z | Along [100] p11m a' = bp b' = c Origin at x, 0, 0 | Along [010] p1g1 a' = c b' = ap Origin at 0, y, 0 |
Maximal non-isomorphic subgroups
| I | [2] P1 (1) | 1 |
| IIa | none |
| IIb | [2] A11a (b' = 2b, c' = 2c) (Cc, 9) |
Maximal isomorphic subgroups of lowest index
| IIc | [2] P11a (c' = 2c) (Pc, 7); [2] P11a (b' = 2b or a' = a + 2b, b' = 2b) (Pc, 7) |
Minimal non-isomorphic supergroups
| I | [2] P2/c (13); [2] P21/c (14); [2] Pmc21 (26); [2] Pcc2 (27); [2] Pma2 (28); [2] Pca21 (29); [2] Pnc2 (30); [2] Pmn21 (31); [2] Pba2 (32); [2] Pna21 (33); [2] Pnn2 (34); [2] Aem2 (39); [2] Aea2 (41) |
| II | [2] A11a (Cc, 9); [2] B11m (Cm, 8); [2] I11a (Cc, 9); [2] P11m (a' = 1/2a) (Pm, 6) |
UNIQUE AXIS c, DIFFERENT CELL CHOICES
P11a
UNIQUE AXIS c, CELL CHOICE 1
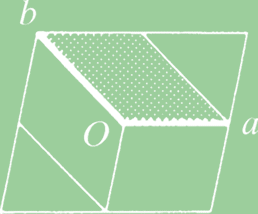
Origin on glide plane a
| Asymmetric unit | 0 ≤ x ≤ 1; 0 ≤ y ≤ 1; 0 ≤ z ≤ 1/2 |
Generators selected (1); t(1, 0, 0); t(0, 1, 0); t(0, 0, 1); (2)
Positions
| Multiplicity, Wyckoff letter, Site symmetry | Coordinates | Reflection conditions | |||||
| General: | |||||||
|
| hk0 : h = 2n h00 : h = 2n |
P11n
UNIQUE AXIS c, CELL CHOICE 2
Origin on glide plane n
| Asymmetric unit | 0 ≤ x ≤ 1; 0 ≤ y ≤ 1; 0 ≤ z ≤ 1/2 |
Generators selected (1); t(1, 0, 0); t(0, 1, 0); t(0, 0, 1); (2)
Positions
| Multiplicity, Wyckoff letter, Site symmetry | Coordinates | Reflection conditions | |||||
| General: | |||||||
|
| hk0 : h + k = 2n h00 : h = 2n 0k0 : k = 2n |
P11b
UNIQUE AXIS c, CELL CHOICE 3
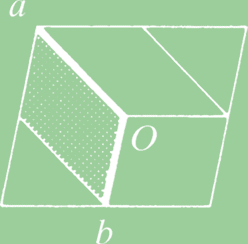
Origin on glide plane b
| Asymmetric unit | 0 ≤ x ≤ 1; 0 ≤ y ≤ 1; 0 ≤ z ≤ 1/2 |
Generators selected (1); t(1, 0, 0); t(0, 1, 0); t(0, 0, 1); (2)
Positions
| Multiplicity, Wyckoff letter, Site symmetry | Coordinates | Reflection conditions | |||||
| General: | |||||||
|
| hk0 : k = 2n 0k0 : k = 2n |
