P-4m2 D2d5 -4m2 Tetragonal info
No. 115 P-4m2 Patterson symmetry P4/mmm

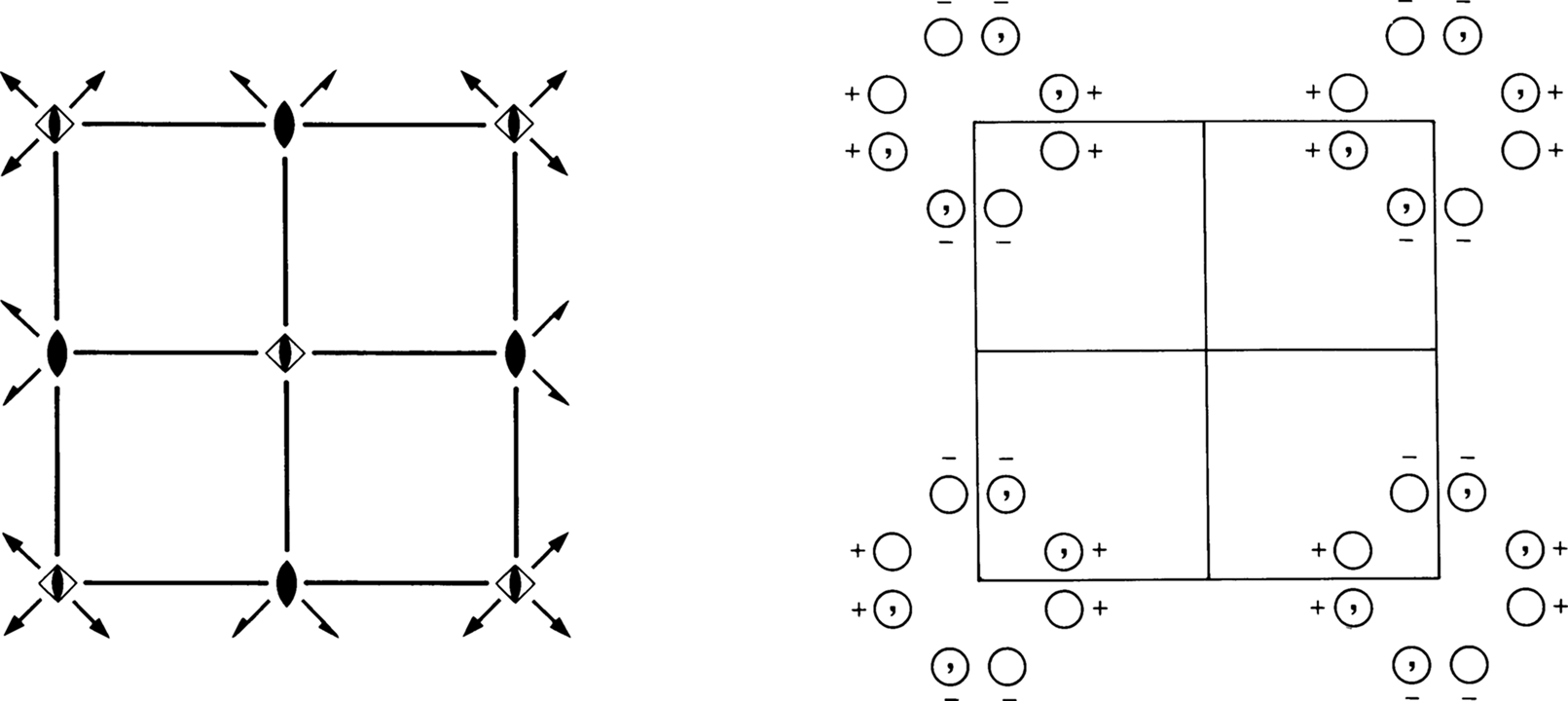
symmetry group diagram

Origin at -4m 2

Asymmetric unit 0 ≤ x ≤ 1/2; 0 ≤ y ≤ 1/2; 0 ≤ z ≤ 1/2

Symmetry operations

(1)  1   (2)  2   0, 0, z(3)  -4+   0, 0, z; 0, 0, 0(4)  -4-   0, 0, z; 0, 0, 0
(5)  m   x, 0, z(6)  m   0, y, z(7)  2   x, x, 0(8)  2   x, -x, 0

Generators selected (1); t(1, 0, 0); t(0, 1, 0); t(0, 0, 1); (2); (3); (5)

Positions

Multiplicity, Wyckoff letter,
Site symmetry		 Coordinates Reflection conditions
 General:
8 l 1
(1) x, y, z(2) -x, -y, z(3) y, -x, -z(4) -y, x, -z
(5) x, -y, z(6) -x, y, z(7) y, x, -z(8) -y, -x, -z
no conditions
    Special: as above, plus
4 k  . m . 
x, 1/2, z -x, 1/2, z 1/2, -x, -z 1/2, x, -z
no extra conditions
4 j  . m . 
x, 0, z -x, 0, z 0, -x, -z 0, x, -z
no extra conditions
4 i  . . 2 
x, x, 1/2 -x, -x, 1/2x, -x, 1/2 -x, x, 1/2
no extra conditions
4 h  . . 2 
x, x, 0 -x, -x, 0x, -x, 0 -x, x, 0
no extra conditions
2 g  2 m m . 
0, 1/2, z 1/2, 0, -z
hk0 : h + k = 2n
2 f  2 m m . 
1/2, 1/2, z 1/2, 1/2, -z
no extra conditions
2 e  2 m m . 
0, 0, z 0, 0, -z
no extra conditions
1 d  -4 m 2 
0, 0, 1/2
no extra conditions
1 c  -4 m 2 
1/2, 1/2, 1/2
no extra conditions
1 b  -4 m 2 
1/2, 1/2, 0
no extra conditions
1 a  -4 m 2 
0, 0, 0
no extra conditions

Symmetry of special projections

Along [001]   p4mm
a' = a   b' = b   
Origin at 0, 0, z		Along [100]   p1m1
a' = b   b' = c   
Origin at x, 0, 0		Along [110]   p2mm
a' = 1/2(-a + b)   b' = c   
Origin at x, x, 0

Maximal non-isomorphic subgroups

I [2] P-411 (P-4, 81)1; 2; 3; 4
  [2] P2m1 (Pmm2, 25)1; 2; 5; 6
  [2] P212 (C222, 21)1; 2; 7; 8
IIa none
IIb[2] P-4c2 (c' = 2c) (116); [2] C-4m21 (a' = 2a, b' = 2b) (P-421m, 113); [2] C-4m2 (a' = 2a, b' = 2b) (P-42m, 111); [2] F-4m2 (a' = 2a, b' = 2b, c' = 2c) (I-42m, 121)

Maximal isomorphic subgroups of lowest index

IIc[2] P-4m2 (c' = 2c) (115); [9] P-4m2 (a' = 3a, b' = 3b) (115)

Minimal non-isomorphic supergroups

I[2] P4/mmm (123); [2] P4/nmm (129); [2] P42/mmc (131); [2] P42/nmc (137)
II[2] C-4m2 (P-42m, 111); [2] I-4m2 (119)








































to end of page
to top of page